Ideal infield moisture 💧

Evan C. Mascitti

2021-05-23

Craig Sampsell asked an interesting question on Twitter recently:

“Has anyone looked into the ideal saturation percentage for an infield skin?”

[edit, 2024: Unfortunately, Craig seems to have taken down his twitter page; thus, I can’t link directly to the post.]

Craig’s tweet caught my attention because I think about this topic quite a bit. I didn’t think I could distill my thoughts into a single tweet, so I wrote this post instead. I’ll take a stab at the calculation and describe my general views on using data to manage infield water content.

But first, some terminology
.

I am going to give my answer/opinion to Craig’s question below. However, we first need to define some terms so we are all speaking the same language.1

Moisture vs behavior

We groundskeepers often talk about “water content” or “moisture” in a philosophical way. In the case of Figure 1 we might say:

“The dirt has really nice moisture today. It’s firm underfoot but I could still push a key all the way down.”

The canonical "key test" for checking the consistency of an infield

Figure 1: The canonical “key test” for checking the consistency of an infield

We use the term “moisture” casually, but we are really describing soil behavior. Soil behavior is a term that describes what happens when you apply a force to the soil How does the soil behave? Does it offer resistance or does it easily give way? Can you it deform into a new shape? Will it stick to metal tools?

Two main types of behavior are brittle and ductile. Brittle behavior means the soil breaks into multiple pieces when you apply a force. Ductile behavior means the soil will deform into a new shape. These are really important on the infield. A brittle infield will be chunky and full of pockmarks. A ductile surface means the cleats won’t tear any soil out and the ball will bounce true, even if it hits a small cleat dimple. Ductility and the corkboard effect go hand-in-hand.

“Hold on,” you might think, “we don’t need all that confusing jargon. I manage moisture by feel. I can see it with my eyes and feel it with my feet.”

I completely agree that we can manage moisture intuitively without defining all kinds of language and calculations. There’s no substitute for experience, observation, and common sense. And just to be totally clear: moisture is definitely the main thing driving soil behavior.

My point is this: although they are closely related, water content and soil behavior are two separate concepts. Water content is a number, plain and simple. Behavior is a squishier concept (bad soil pun! 🙄) but it can be measured too.

Here is an example to drive the point home: imagine your infield has volumetric water content of 10% and your sand rootzone also has volumetric water content of 10%. They have the same water content, but their behavior will be completely different. The infield will be like concrete, while the rootzone will be more forgiving but pretty unstable. Water content values are not directly comparable for different soils. This is for 2 reasons:

  1. The texture of the soil greatly changes how the soil will behave at a given water content number.
  2. Even if the textures are similar, the soils might have different porosity, which changes the amount of water needed to fill the soil’s voids.

Effective saturation

Back to the original question - what is the “ideal” saturation percentage? Obviously there’s a degree of subjectivity around what is “ideal” - but I think most groundskeepers would agree that the ideal infield behavior is no chunk-outs and footing that is firm – but not too firm – with low, consistent ball bounces. Dr. McNitt and I recently wrote an article in SportsField Managment Magazine about this topic.

We will stick with volumetric water content (abbreviated \(\theta\)) for this discussion because it is easier to draw and visualize.2

I must make one more pedantic distinction. I would consider “saturation percentage” to be what soil scientists call effective saturation, abbreviated \(S_e\). This is not the same as water content. \(S_e\) is the fraction of the soil pores which are filled with water. If the soil is completely saturated (no air whatsoever), \(S_e=1\) and if the soil is completely dry \(S_e=0\). If half the pores are full, \(S_e=\text{0.5}\). Pretty simple.

I think Craig’s question was actually about water content, not effective saturation. [Craig, if you are reading this, feel free to correct me.] For water content, the answer is “it depends,” for the reasons discussed below. I am going to answer first for effective saturation \(S_e\) and then we will calculate the water content \(\theta\).

I think the ideal degree of infield saturation is
.. fully saturated!! I know what you’re thinking.

“Are you nuts?!?!!? The infield is like toothpaste when it’s saturated!”

That’s not the degree of saturation I am talking about. I am going to show this with a calculation in a minute, but let’s think the idea through first.

Saturation only means that the pores are completely filled with water. It doesn’t necessarily mean the soil will be soup. The surfaces of clay particles have a really high affinity for water. They love water so much that in a sense, they are engaged in a ‘tug-of-war’ for the individual H2O molecules. For every force there is an equal and opposite force (Newton’ 3rd law of motion). That means if the particles are pulling on the water, then the water is also pulling on the particles. Stop reading and chew on that idea for a moment.

Since nobody wants to let go, the particles are pulled together and the friction between them provides enough resistance that we can play baseball. As groundskeepers this is the thin margin where we like to live - wet enough to get the cleat-in, cleat-out effect with nice ball bounce
 but not so wet that the surface is soft and dangerous. In microscopic terms the capillary forces are weak enough to let the particles slide past one another, but strong enough to limit the total movement. Basically, the old adage about water acting as “glue” is right on.

Figure 2 shows an exaggerated diagram of how this happens. Once the soil is saturated, more water might still be added (intentionally with a hose, or unintentionally during a rain game). The platy clay particles get separated a little further away from each other, and the water isn’t being tugged as strongly as before. This makes the particles easier to move. With further water additions the configuration of the grains becomes so loose that the particles slide right by each other with virtually no resistance. This situation is a quagmire and it’s why high-maintenance fields have a tarp.

**Left**: Soil is _just_ saturated; moderate friction between clay prevents slippage. **Right**: Soil remains saturated; further increase in water content promotes easy sliding of platy clay particles.

Figure 2: Left: Soil is just saturated; moderate friction between clay prevents slippage. Right: Soil remains saturated; further increase in water content promotes easy sliding of platy clay particles.

Here, the water content has increased, but \(S_e\) hasn’t - it’s still 100%, just like it was right at the instant the pores became full. The difference is that the additional water has shoved the particles further apart, slightly increasing the total volume of the soil. The extra space is completely filled by the extra water.

On the dry end, if we take away some water and introduce air back into the pores, the network of water is no longer continuous. This makes it easy for cracks to propagate along the voids and break the soil into pieces - i.e. chunk-outs.

In reality, no soil at the surface will ever be truly, saturated. This is because it is next to impossible to evacuate all the air from an infield soil
..most soils, even when very wet, contain some entrapped air bubbles and their \(S_e\) value will not exceed 0.9 (90%). However, the \(S_e\) of 1 concept is still useful.

Finally, some numbers

After all this arm-waving, what is the number to target? As I argued above, we are shooting for effective saturation approaching 1. But we can’t directly measure \(S_e\) with a moisture probe. We need an actual volumetric water content (abbreviated \(\theta\)).

Choosing a target water content based on porosity

Let’s assume the infield is compacted to 2.0 \(\mathrm{\frac{g}{cm^3}}\). This is a nice round number and a pretty typical density for a compacted infield mix having 60-70% sand.

We can easily calculate the total porosity of this soil with the formula:

\[\mathrm{porosity = \quad total~volume-\left(\frac{bulk~density}{particle~density}\right)} = \quad 1 - \mathrm{\left( \frac{\rho_{~bulk}}{\rho_{~particle}} \right) }\]

We will assume a typical particle density of 2.7 \(\mathrm{\frac{g}{cm^-3}}\) . Plugging in our numbers, we get the following:

\[\require{cancel} \mathrm{porosity = \quad 1-\frac{2.0~ \cancel{\frac{g}{cm^3}}}{2.7 \cancel{\frac{g}{cm^3}}}} = \quad 0.259 \approx 26~\% \]

So if this were my infield I would shoot for a water content a little above 26%. That would be enough to just fill all the pores.

We can illustrate the pore volumes using a phase diagram (Figure 3). This drawing is not a soil profile - it’s a volumetric representation of what you’d see if we could separate a soil out into its pure components. Kind of like those density jars you made in 6th grade science class. Anyway, this soil is 70% sand by mass and it is packed to a bulk density of 2.0 \(\mathrm{\frac{g}{cm^3}}\). By volume, the soil is 74% solids and 26% pores (summing to 100%). The 26% porosity comprises 18% water and 8% air, for an \(S_e\) value of \(0.18/0.26=0.69\).

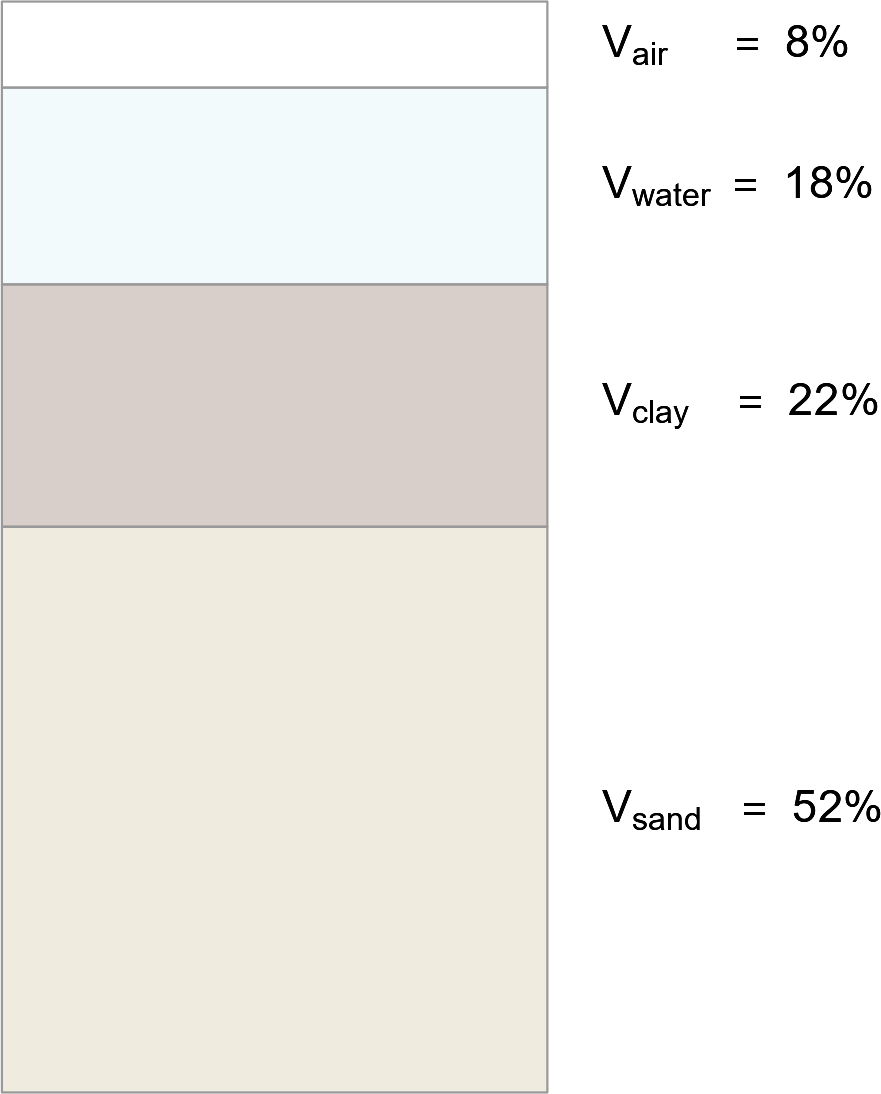
Example phase diagram showing volume proportions of solid phases plus water and air.

Figure 3: Example phase diagram showing volume proportions of solid phases plus water and air.

To calculate porosity and \(S_e\) we need to know the soil’s bulk density and its water content. Measuring bulk density of your infield is easy enough to do with some PVC pipe and a kitchen scale, but I will save that for another post.

Choosing the target water content by feel

I think a more realistic way to pick a target number for \(\theta\) circles back to my soapbox about soil behavior. Experiment with a heavy watering on your infield - take a reading when you think it’s just on the verge of becoming unplayable. That’s your upper limit. Repeat the measurement as the soil dries, and get a sense for when you think the field plays the best. That’s your target. In a sense this raises the question of why you would even need the number in the first place, if you are going to just use feel to decide how much to water. Tom Burns captured this perfectly:

I completely agree with Tom. If you’re already an exert on the hose and you know your infield well, I don’t think having a number will dramatically improve your watering practices.

I think what Craig was suggesting is that giving a number to a new employee could be useful if they haven’t yet developed the “feel” for how much water to add [again, unfortunately his follow-up post is no longer available]

I think another potential use of the maximum water content could be having something to show your higher-ups about why the field has to be closed today. If the water content is above the maximum, players’ safety could be at risk, and you could do significant damage to the infield. As a groundskeeper you already know this, of course, and you don’t really need the number to decide if the field is safe - but bosses like numbers, and they help explain why you made a particular choice.

How much to water?

Assuming we’ve chosen a target water content, we can go one step further. How much water should we add in order to raise the the water content to our target level?

Continuing with the example above, our target \(\theta\) is 26% in the top 2” of soil and we are currently at 18%. These numbers are just an example: other soils could have a different target \(\theta\).

Say we have a 1” hose that can deliver 32 gallons per minute.3

How long do we need to water?

A 2 inch soil depth is \(2/12=0.167\) ft, so I first calculated the volume of soil “cavity” we are trying to affect, then multiplied by our 8% increase:

\[\require{cancel}\mathrm{ \frac{0.167~ft}{} \times \frac{10,000~{ft^2}}{1~infield} \times 0.08= \frac{133.3~ft^3~H_2O}{1~infield}}\]

Now we can convert the water volume to gallons and then to time, based on the hose flow rate:

\[\require{cancel}\mathrm{\frac{133.3~\cancel{ft^3~H_2O}}{1~infield} \times \frac{7.48~\cancel{gal~H_2O}}{1~\cancel{ft^3~H_2O}} \times \frac{1~minute}{32~\cancel{gal~H_2O}} = 31.2~minutes}\]

For a dry infield, that seems like a reasonable watering time to me.

Also note that we haven’t factored in any evaporation loss, so we would need to add even more water to offset that expected evaporation. Obviously, the rate of loss will vary based on weather, but in my experience a well-watered infield left alone on a sunny day will lose about 1-2% \(\theta\) per hour in the top 2”.

If the infield had been sitting for several days without rain or hand-watering, its water content would be considerably less. \(\theta\) will never get down to 0% but if it dropped to 2%, we would need to add 500 ft3 - requiring us to water for an hour and a half to get the desired 24% bump. And that’s assuming every drop gets into the profile, which it definitely won’t – some will evaporate first. To those new to watering the infield, it can be pretty shocking to add so much water.

It’s pretty unlikely anyone could water for this long without most of the water just running off.4 If you have any baseball experience you know it takes several heavy waterings spaced over a couple days to fully re-hydrate the profile.

Summary

These “back-of-the napkin” calculations are not a substitute for paying attention and learning how to hand-water by feel. Fine adjustments are always made based on instinct, experience and weather forecasts. It is still fun to play with the numbers, especially when they agree with our experience and intuition. And a target water content could be useful for less-experienced employees learning the ropes. Thanks to Craig for bringing this topic up, and to Ryan DeMay for tagging me on the thread!

Here are three summary points to close:

Happy hosing! 💧


  1. This is definitely my ‘book-boy’ side speaking, not my ‘dirt-raker’ side. Words only have meaning when they are tied to the right ideas. In my experience, a lot of disagreements boil down to people having different concepts of the same words, even though they actually believe the same thing.↩

  2. There is another way to measure water content - gravimetric - which is considered more “absolute” in lab work.↩

  3. Do not assume that the flow rate listed on your hoze nozzle is correct. These are normally labeled between 30 and 60 gpm, but the actual delivery rate will depend highly on your water pressure. I recommend calibrating your hose/nozzle by using it to fill up a large trash can of known volume and recording the time. For example, if it takes 94 seconds to fill up a 50 gallon tub, your flow rate is 32 gpm: \[\require{cancel}\mathrm{\frac{50~gal}{94~\cancel{sec}} \times \frac{60~\cancel{sec}}{1~min} \approx \frac{32~gal}{1~min}}\] ↩

  4. If you can, your mix is way too sandy.↩